Relation with monomials Given a monomial equation =, taking the logarithm of the equation (with any base) yields = Setting = and = , which corresponds to using a log–log graph, yields the equation = where m = k is the slope of the line and b = log a is the intercept on the (log y)axis, meaning where log x = 0, so, reversing the logs, a is the y value corresponding The graph of #y=x^26xk# as its vertex on the xaxis How do you find the value of k?Try varying the values of a and k and examine what effects this has on the graph
1
Y=k/x^2 graph
Y=k/x^2 graph-Use different colors to graph the family of graphs defined by y = k x 2, where k ∈ {1, 1 2, 1 3, 1 4} What happens to the graph when the denominator of k is very large?Y=kx^2 Author Mr Friend The graph shows Use the buttons to change the value of k k positive smile k negative frown Taking a point from the graph it is possible to work backwards to determine the value of k New Resources Fraction Addition;




Non Linear Further Maths U3 4
Power Function Graph y=a/x^2 Author KS Wong Topic Function Graph Instructions 1 Move the slider to the left or the right 2 Observe the SHAPE and EQUATION of the graph as a changes The usual approach uses the notation y = f (x h) k To move the graph 3 units right and 2 units up, we would have y = f (x 3) 2 Again, students wonder why the upward shift of 2 is done with 2 while the shift to the right is done with 3 That doesn't seem consistent, either Station 2 The Graph of y= kx^2 Observe the overall view of the graph, use the slider to change the value of k (noting quadrants and trends of the graph) and record all findings as responses to the questions on the lab sheet
Yes it is indeed Given, xy= k, If you try putting either x=0 or y=0 then you will find out that k=0 which is not given in question (I assume k is a nonzero constant, if it is 0 then the curve xy=0 will actually represent either x =0 Ie yz planThese graphs are symmetrical about the yaxis n = 2, y = k / x^2 When n = 2, the graph produced is called a truncus A truncus also has asymptotes n = 3, y = kx^3 This is a cubic graph where n = 3 Varying k values dilates the graph, and a negative k value reflects it Linear representation of nonlinear relationsY = a(x h) 2 Vertex Form k k vertical translation 'k' units k > 0 , the graph is translated 'k' units up k< 0 , the graph is translated 'k' units down y = x 2 4 y = x 2
Algebra Graph y=x^ (1/2) y = x1 2 y = x 1 2 Graph y = x1 2 y = x 1 2X≠0 The range is all real numbers;Function Grapher is a full featured Graphing Utility that supports graphing up to 5 functions together You can also save your work as a URL (website link) Usage To plot a function just type it into the function box Use "x" as the variable like this Examples sin(x) 2x−3;



All Elementary Mathematics Study Guide Functions And Graphs Elementary Functions And Their Graphs
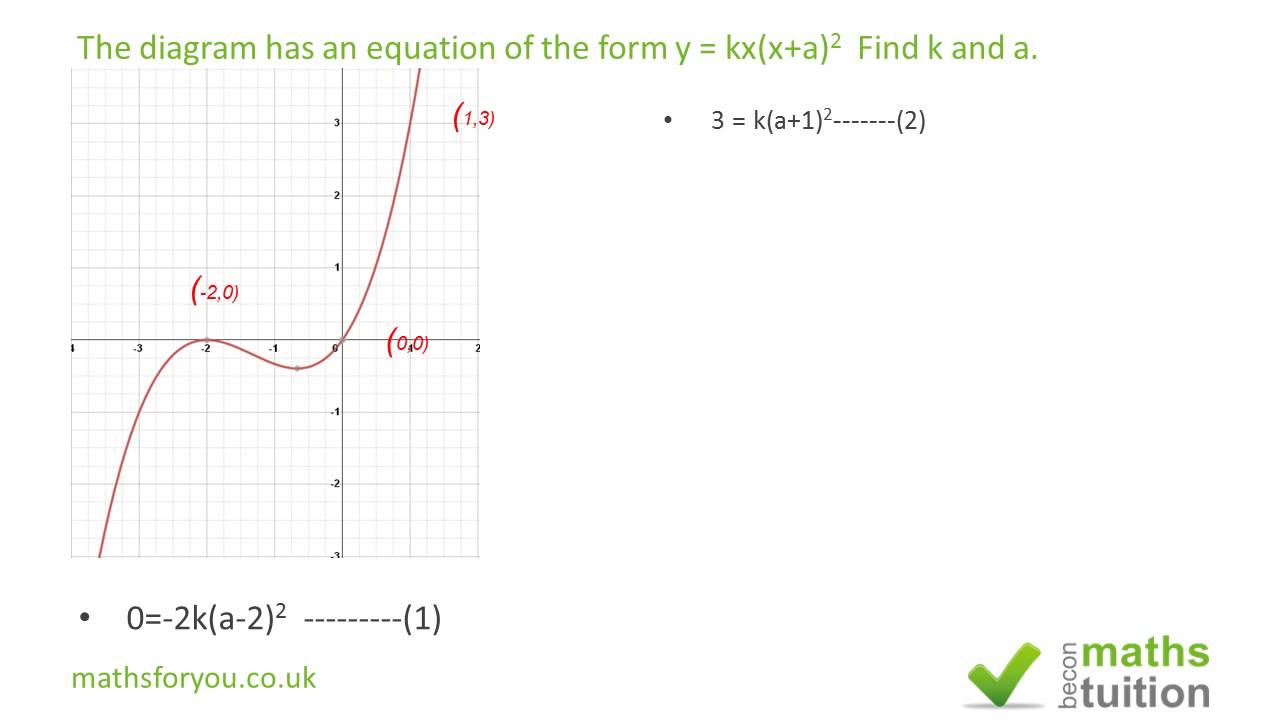



Simultaneous Graph Equation Y Kx X A 2 Find K And A Youtube
Y = 2 −x To graph a general exponential function in the form, y = abx − h k begin by sketching the graph of y = abx and t hen translate the graph horizontally by h units and vertically by k units Example Graph 3 (2 x−1) − 4 SolutionSelect one a 3/4If x = 5, y = 2(5) 6 = 4 We then note that the graph of (5, 4) also lies on the line To find solutions to an equation, as we have noted it is often easiest to first solve explicitly for y in terms of x Example 2 Graph x 2y = 4 Solution We first solve for y in terms of x to get We now select any two values of x to find the associated




Non Linear Further Maths U3 4



Http Www Ia Maths Co Uk Site Wp Content Uploads 14 06 Quadratics Notes Pdf
Since y^2 = x − 2 is a relation (has more than 1 yvalue for each xvalue) and not a function (which has a maximum of 1 yvalue for each xvalue), we need to split it into 2 separate functions and graph them together So the first one will be y 1 = √ (x − 2) and the second one is y 2 = −√ (x − 2) For Examples 3 & 4, graph the rational functions Find the zero, yintercept, asymptotes, domain and range Example 3 y = 4 x − 2 Solution There is no yintercept because the yaxis is an asymptote The other asymptote is y=−2 The domain is all real numbers; Graph transformations Given the graph of a common function, (such as a simple polynomial, quadratic or trig function) you should be able to draw the graph of its related function



Past Papers Solutions Cambridge International Examinations Cie As A Level Mathematics 9709 Pure Mathematics 2 P2 9709 02 Year 16 Oct Nov P2 9709 23 Q 2 O A Level Solutionso A Level Solutions



Pplato Basic Mathematics Quadratic Functions And Their Graphs
Free graphing calculator instantly graphs your math problemsThis might feel a bit more difficult to graph, because just about all of my yvalues will be decimal approximationsBut if I round off to a reasonable number of decimal places (one or two is generally fine for the purposes of graphing), then this graph will be fairly easyAnswer to If the parabola of equation y = k x^2 is tangent to the line of the equation y = x, then what is the value of k?
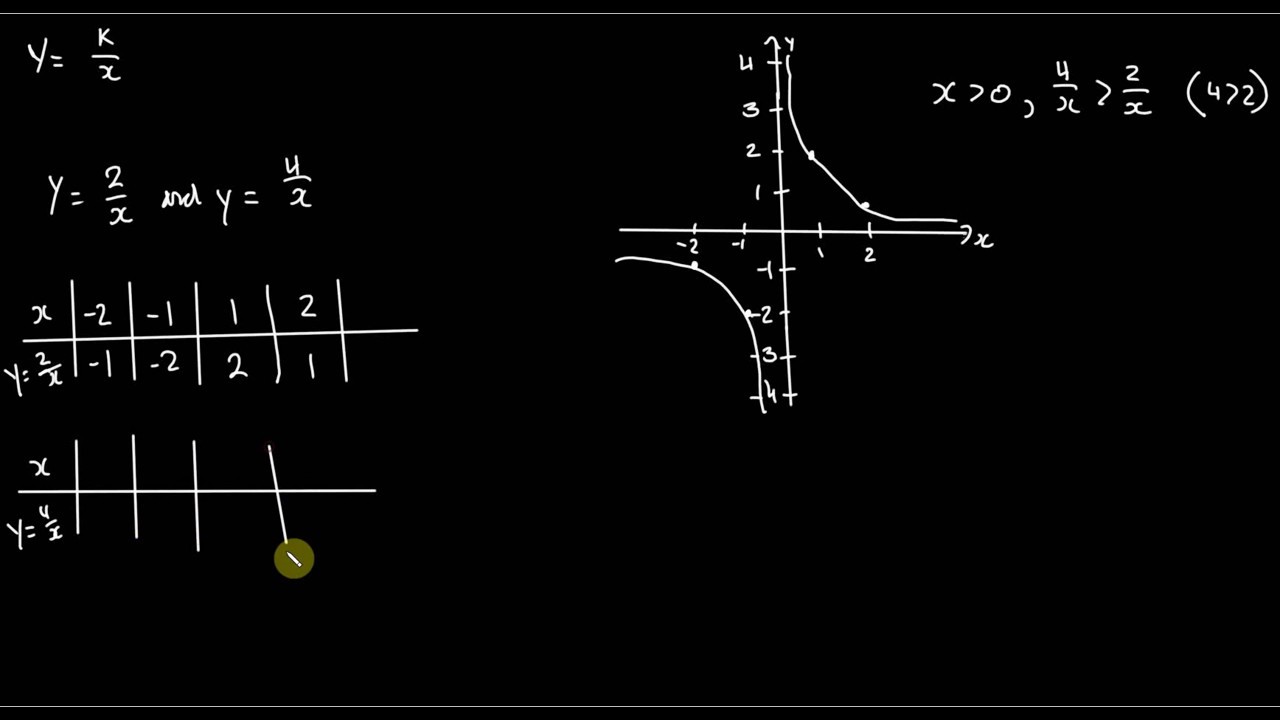



Sketch And Interpret Graphs Of Reciprocal Functions Y K X 2 Youtube
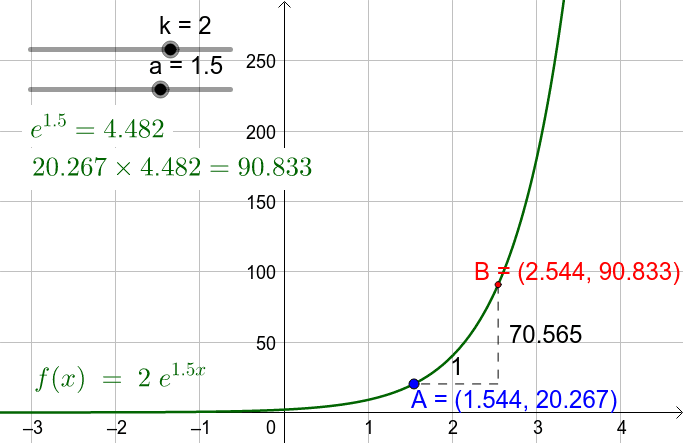



Graph Of Y K E Ax Geogebra
Find the orthogonal trajectories of the family of curves Use a graphing device to draw several members of each family on a common screen x^2 y^2 = kMultiply 1 4 1 4 by 1 1 Add 0 0 and 1 4 1 4 Substitute the values of a a, d d, and e e into the vertex form a ( x d) 2 e a ( x d) 2 e Set y y equal to the new right side Use the vertex form, y = a ( x − h) 2 k y = a ( x h) 2 k, to determine the values of a a, h h, and k kConsider the graph of the parabola y=ax^2 Its vertex is clearly at (0,0) Now, if you replace x with xh in any equation, its graph gets shifted to the right by a distance of h




The Power Functions Direct Variation What Is It
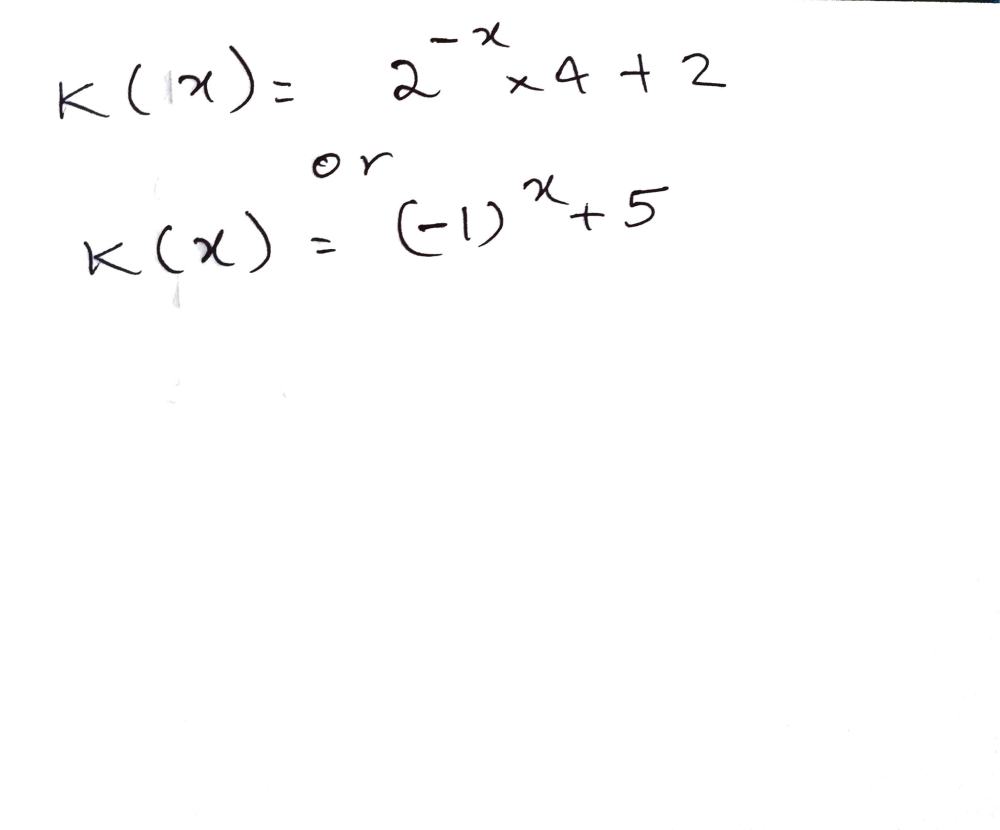



Y 5 1 The Sketch Shows The Graph Of The Function Gauthmath
Share your findings on the discussion boardThe next two examples show how changing y = x^2 to y = x^2k or to y = (xh)^2, respectively, affects the graph of a parabola Example 3 GRAPHING A RELATION OF THE y = x^2k Graph y = x^24 Each value of y will be 4 less than the corresponding value of y = x^2 This means that y = x^24 has the same shape as y = x^2 but is shifted 4 units downGet the free "Circle Grapher (xh)^2 (y k)^2 = r^2" widget for your website, blog, Wordpress, Blogger, or iGoogle Find more Mathematics widgets in WolframAlpha




Write And Graph A Direct Variation Equation Ppt Download



Pplato Basic Mathematics Quadratic Functions And Their Graphs
Sin (x)cos (y)=05 2x−3y=1 cos (x^2)=y (x−3) (x3)=y^2 y=x^2 If you don't include an equals sign, it will assume you mean " =0 " It has not been well tested, so have fun with it, but don't trust it If it gives you problems, let me know Note it may take a few seconds to finish, because it has to do lots of calculationsRevising how to sketch reciprocal graphsGo to http//wwwexamsolutionsnet/ for the index, playlists and more maths videos on curve sketching and other mathWhere the graph intersects the xaxis, x 2 2kx5 must be equal to zero Thus we can answer the equivalent question For what k does x 2 2kx5 = 0 not have a solution?This is now a simpler problem (roots of a quadratic equation) We can apply the common method of considering the discriminant of x 2 2kx5 Using standard quadratic formula notation where in this case a=1, b




Unit Circle Shifted Upwards So It Is Tangent The Graph Of F X X 2 Mathematics Stack Exchange




Quadratic Functions Mathnology
We are given the quadratic function y = f (x) = (x 2)2 For the family of quadratic functions, the parent function is of the form y = f (x) = x2 When graphing quadratic functions, there is a useful form called the vertex form y = f (x) = a(x −h)2 k, where (h,k) is the vertex Data table is given below (both for the parent function andSimple and best practice solution for Yx=k(xy) equation Check how easy it is, and learn it for the future Our solution is simple, and easy to understand,Graphing y = (x h)2 k In the graph of y = x2, the point (0, 0) is called the vertex The vertex is the minimum point in a parabola that opens upward In a parabola that opens downward, the vertex is the maximum point We can graph a parabola with a different vertex Observe the graph of y
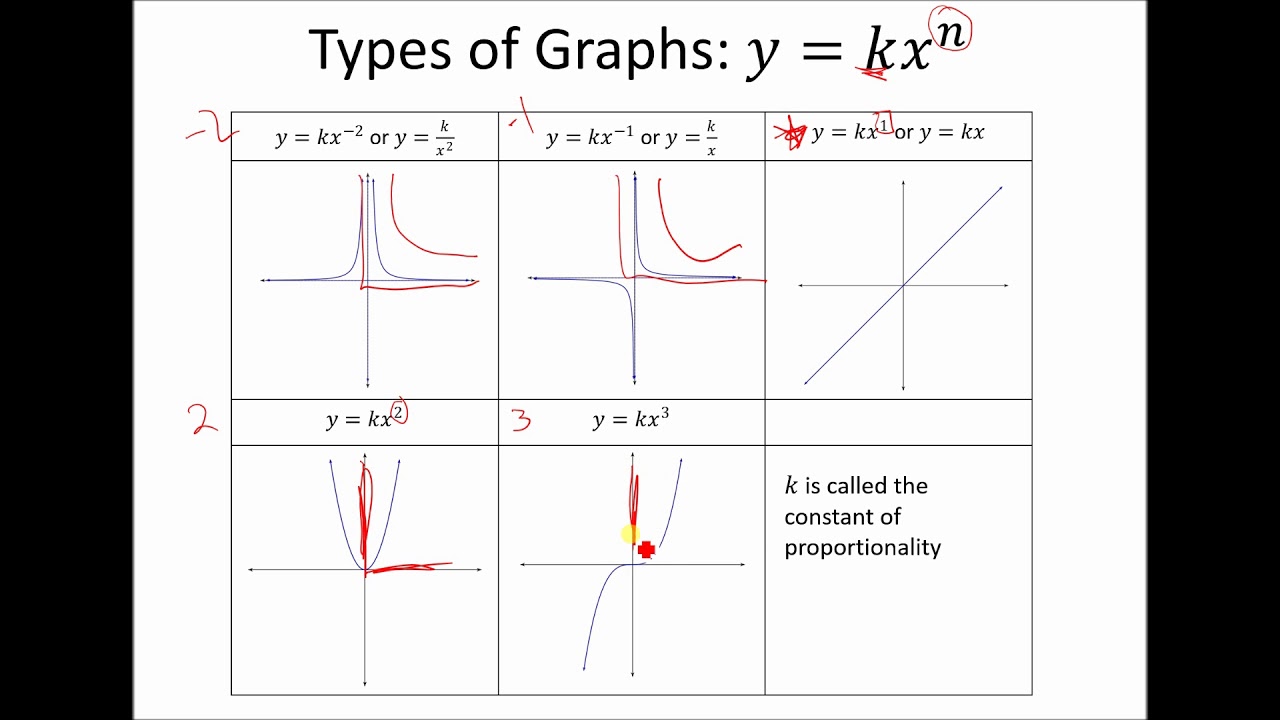



Lesson 7 Constructing Non Linear Graphs Youtube



Why Is The Graph Of Y E Logx Not The Same As Y X Quora
Graph y=x^32 y = x3 − 2 y = x 3 2 Find the point at x = −2 x = 2 Tap for more steps Replace the variable x x with − 2 2 in the expression f ( − 2) = ( − 2) 3 − 2 f ( 2) = ( 2) 3 2 Simplify the result Tap for more steps Raise − 2 2 to the power of 3 3About Press Copyright Contact us Creators Advertise Developers Terms Privacy Policy & Safety How works Test new features Press Copyright Contact us CreatorsGraph y = e x;
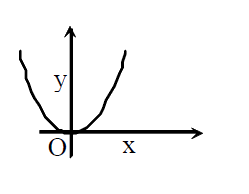



If Y Kx 2 Where K Is Positive Non Zero Constant Then Whi




2 5 The Graph Of Y Kx 2 Youtube
K In this lesson you will learn about graphs of equations of the form y = a ( x − h) 2 k For example, you will look at equations such as y = 3 x 2, y = − 2 x 2, and y = 2 ( x 1) 2 3, and compare them to y = x 2 You will also learn about roots of quadratic equations and how the values of a, h, and k affect the number of rootsGraph y=x2 Find the absolute value vertex In this case, the vertex for is Tap for more steps To find the coordinate of the vertex, set the inside of the absolute value equal to In this case, Add to both sides of the equation Replace the variable with in the expressionSteps to graph x^2 y^2 = 4



Quadratics Graphing Parabolas Sparknotes



Non Linear Graphs In The Form Y Kx N The Engage Wiki
A lesson of the graph y=k/x Blog Aug 10, 21 Preparing for back to school events in a hybrid world; Graph of y=ax^2k Graph of Try varying the values of k and a and see how it affects the graph of this quadratic function Write down what you see as you change each value of 'a' and 'k' Explain why?Cos(x^2) (x−3)(x3) Zooming and Recentering To zoom, use the
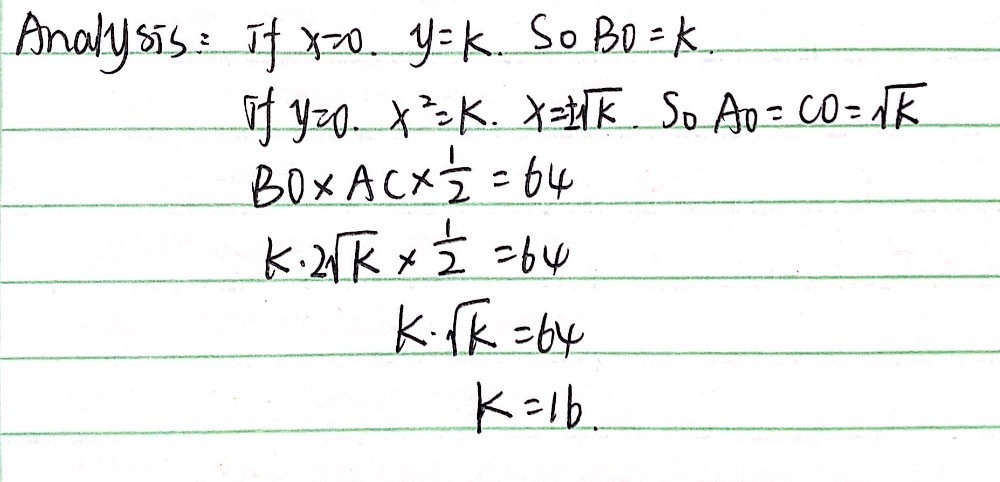



9 The Figure Above Shows The Graph Of Y K X2 Gauthmath
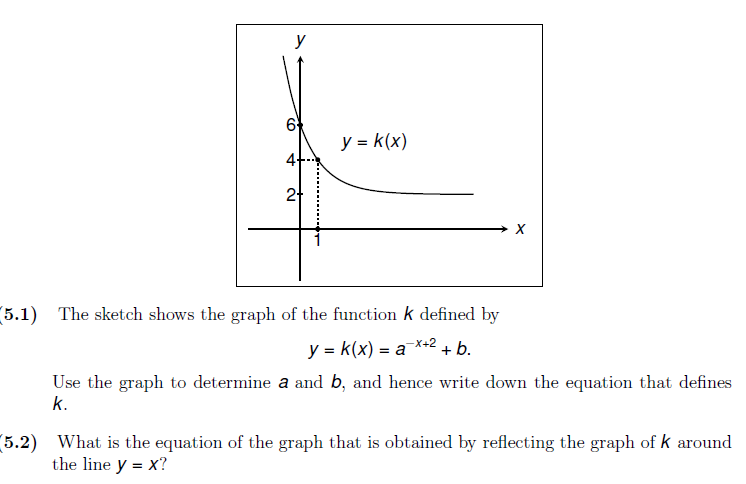



Answered Y 6 Y K X 2 1 The Sketch Shows Bartleby
Any line can be graphed using two points Select two x x values, and plug them into the equation to find the corresponding y y values Tap for more steps Choose 0 0 to substitute in for x x to find the ordered pair Tap for more steps Replace the variable x x with 0 0 in the expression f ( 0) = 0 2 f ( 0) = 0 2Click here👆to get an answer to your question ️ The entire graph of the equation y = x^2 kx x 9 is strictly above the x axis if and only ifExponential graphs are graphs in the form \ (y = k^x\) These graphs increase rapidly in the \ (y\) direction and will never fall below the \ (x\)axis An exponential graph will look like this



Quadratic Functions
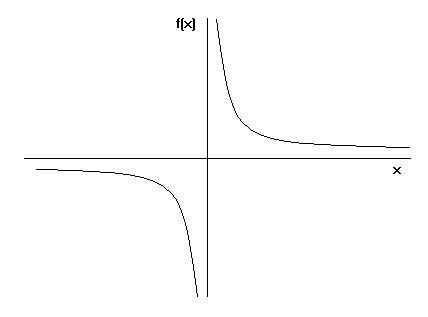



Functions Of A Graph
If we put x = 0, we get z = y2, so the yzplane intersects the surface in a parabola If we put x = k (a constant), we get z = y2 4k2 This means that if we slice the graph with any plane parallel to the yzplane, we obtain a parabola that opens upward Similarly, if y = k, 2the trace is z = 4x k2, which is again a parabola that opens upwardSet y y equal to the new right side y = x 2 y = x 2 y = x 2 y = x 2 Use the vertex form, y = a ( x − h) 2 k y = a ( x h) 2 k, to determine the values of a a, h h, and k k a = 1 a = 1 h = 0 h = 0 k = 0 k = 0 Since the value of a a is positive, the parabola opens up Opens UpAlgebra Graph y=x2 y = x − 2 y = x 2 Use the slopeintercept form to find the slope and yintercept Tap for more steps The slopeintercept form is y = m x b y = m x b, where m m is the slope and b b is the yintercept y = m x b y = m x b Find the values of m m and b b using the form y = m x b y = m x b




Non Linear Further Maths U3 4
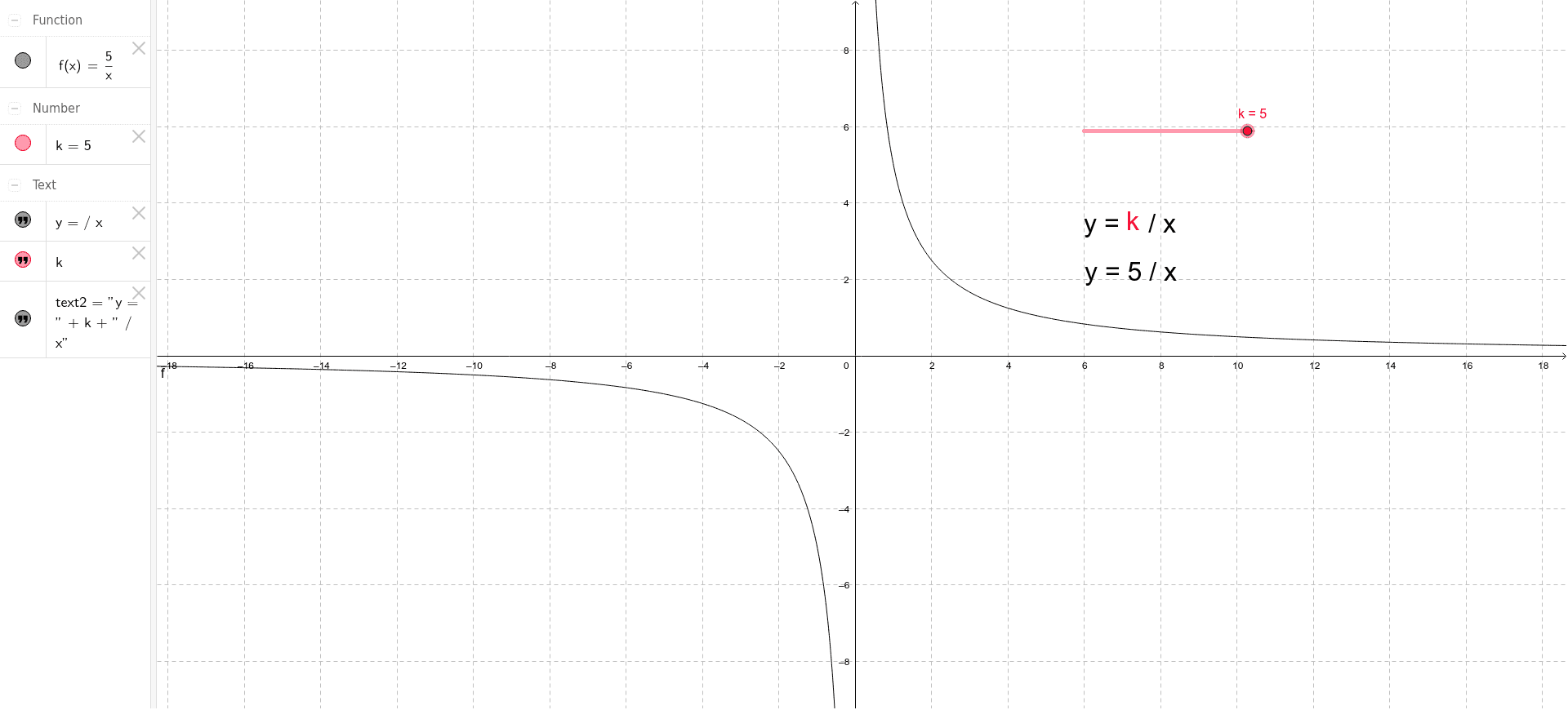



Hyperbola Y K X Geogebra
Graphing Reciprocal Functions – Explanation and Examples Reciprocal functions have the form y= k / x, where k is any real numberTheir graphs have a line of symmetry as well as a horizontal and vertical asymptoteAlgebra Quadratic Equations and Functions Vertex Form of a Quadratic Equation 1 Answer Douglas K Set y = 0, evaluate the quadratic at #h = b/(2a)# and solve for k #k



1
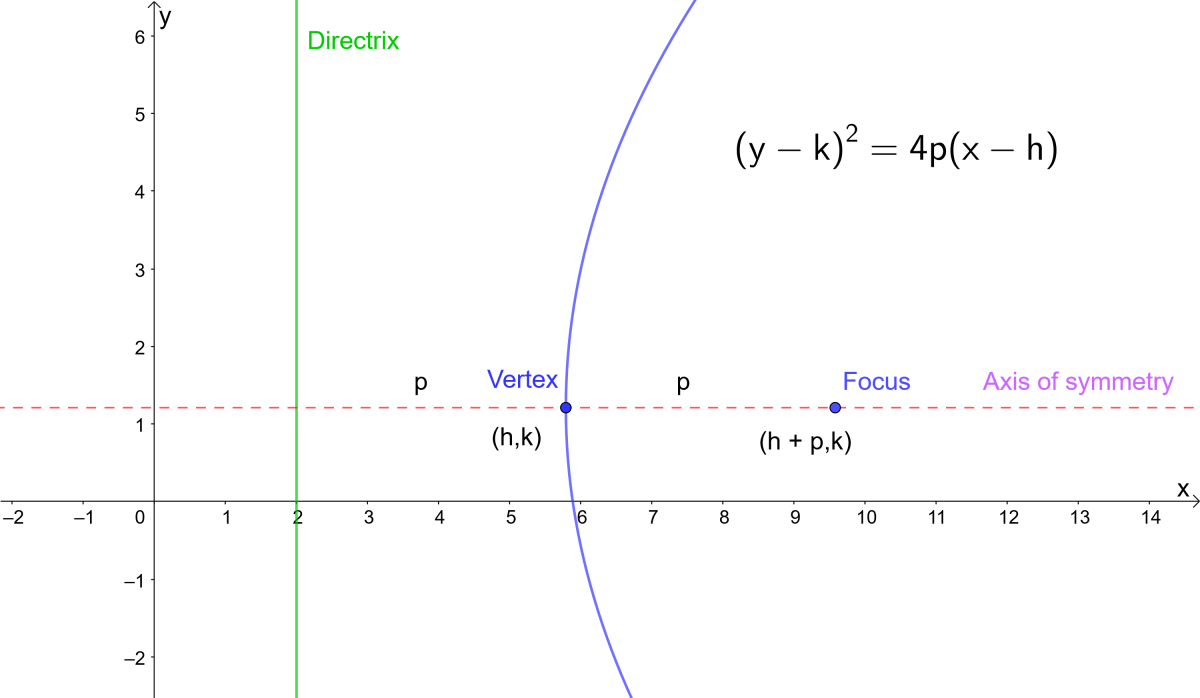



Parabola Equations And Graphs Directrix And Focus And How To Find Roots Of Quadratic Equations Owlcation




Graph The Line Y Kx 1 If It Is Known That The Point M Belongs To It M 1 3 Brainly Com




Fig A8c Linear Line In A Rectilinear Graph Y Kx C Download Scientific Diagram
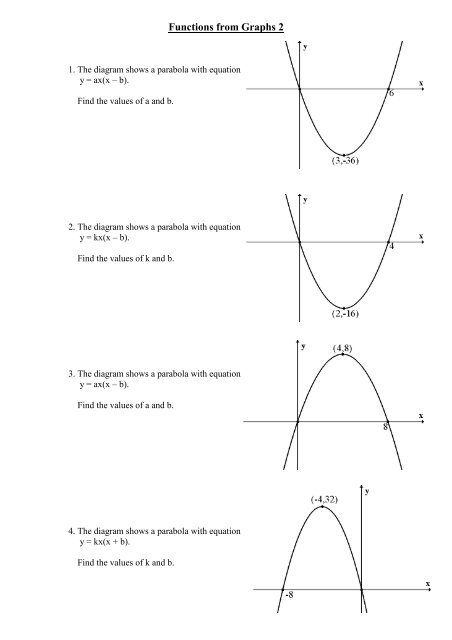



Functions From Graphs 2 Knightswood Secondary School



1
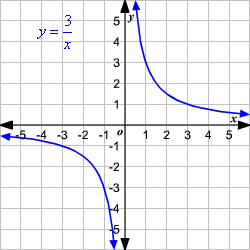



Inverse Variation
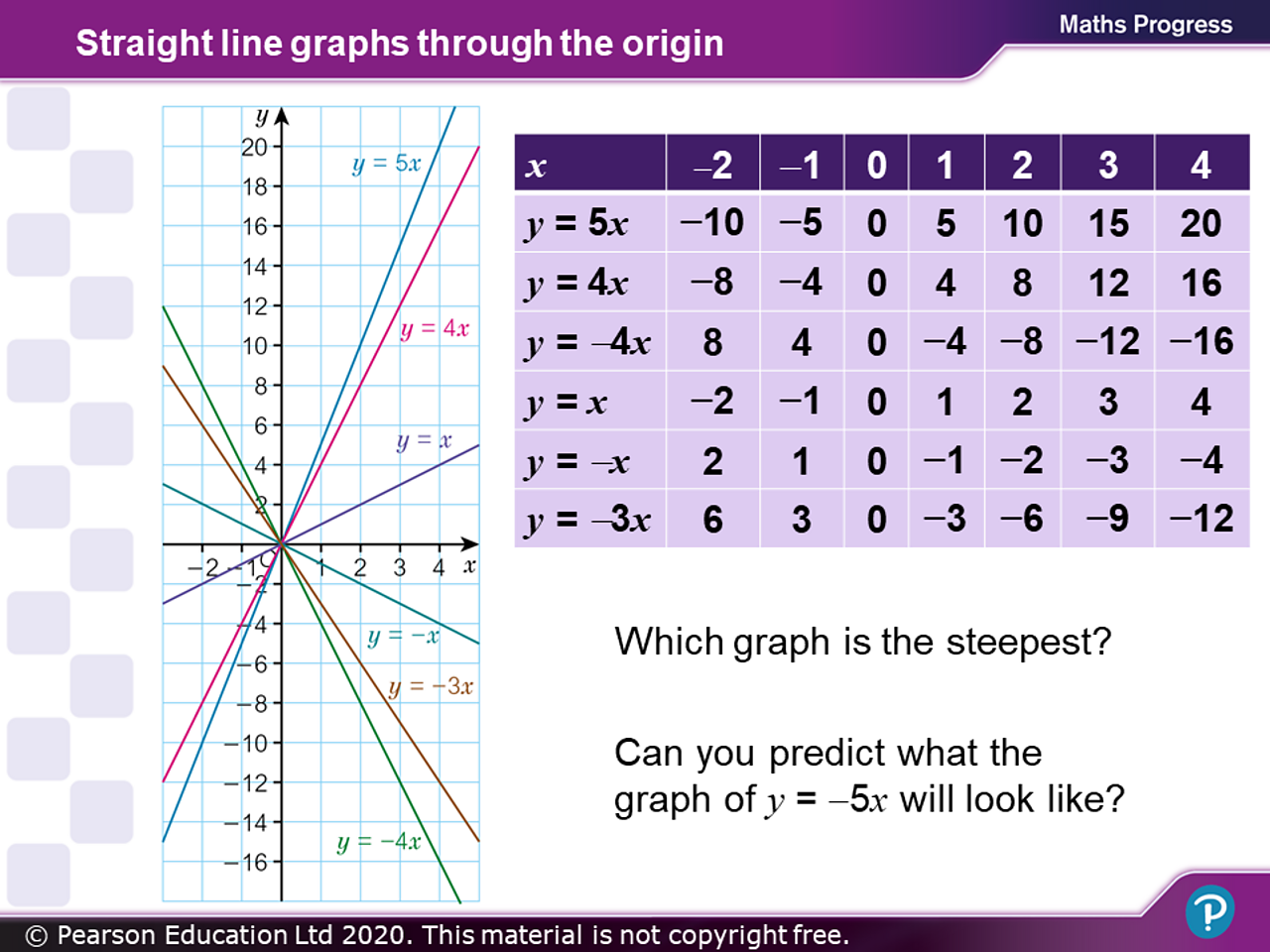



Home Learning With c Bitesize Ks3 Secondary Maths For Year 8 c Bitesize




Chapter 3 1 Variation Ppt Video Online Download
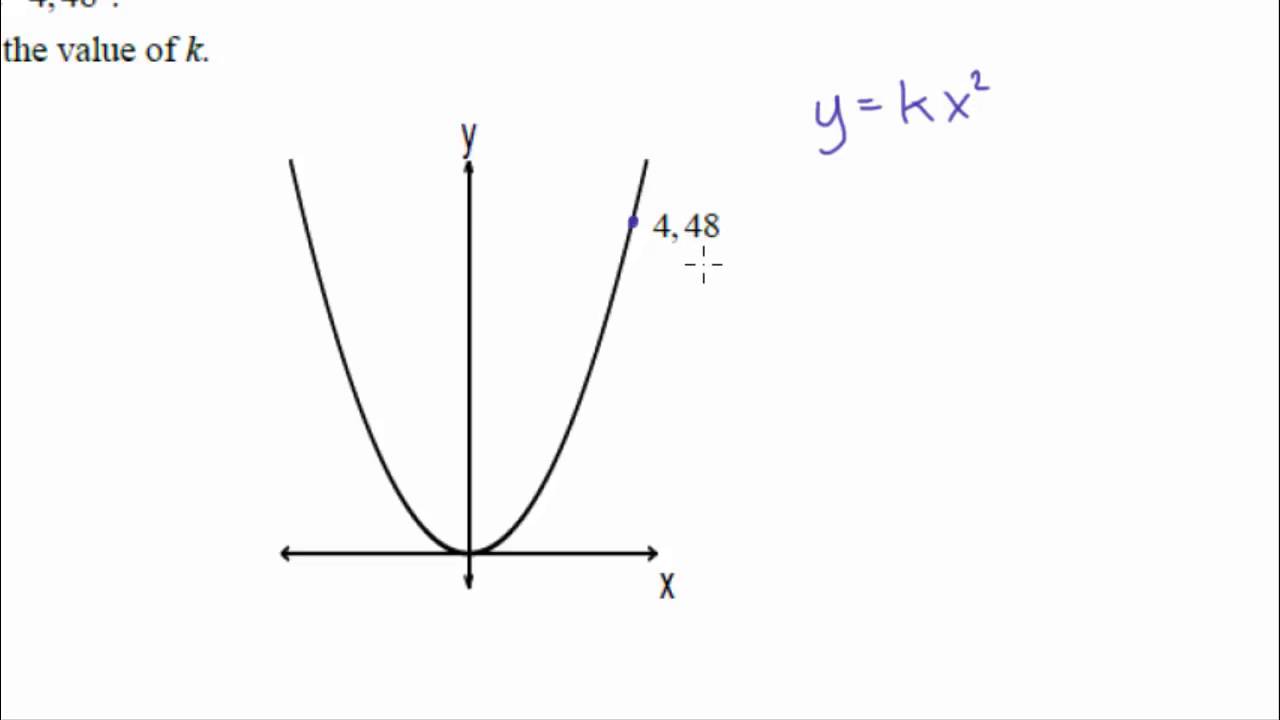



Relationship Nab Practice Quadratic Y Kx 2 Youtube




Y Kx 2 Youtube
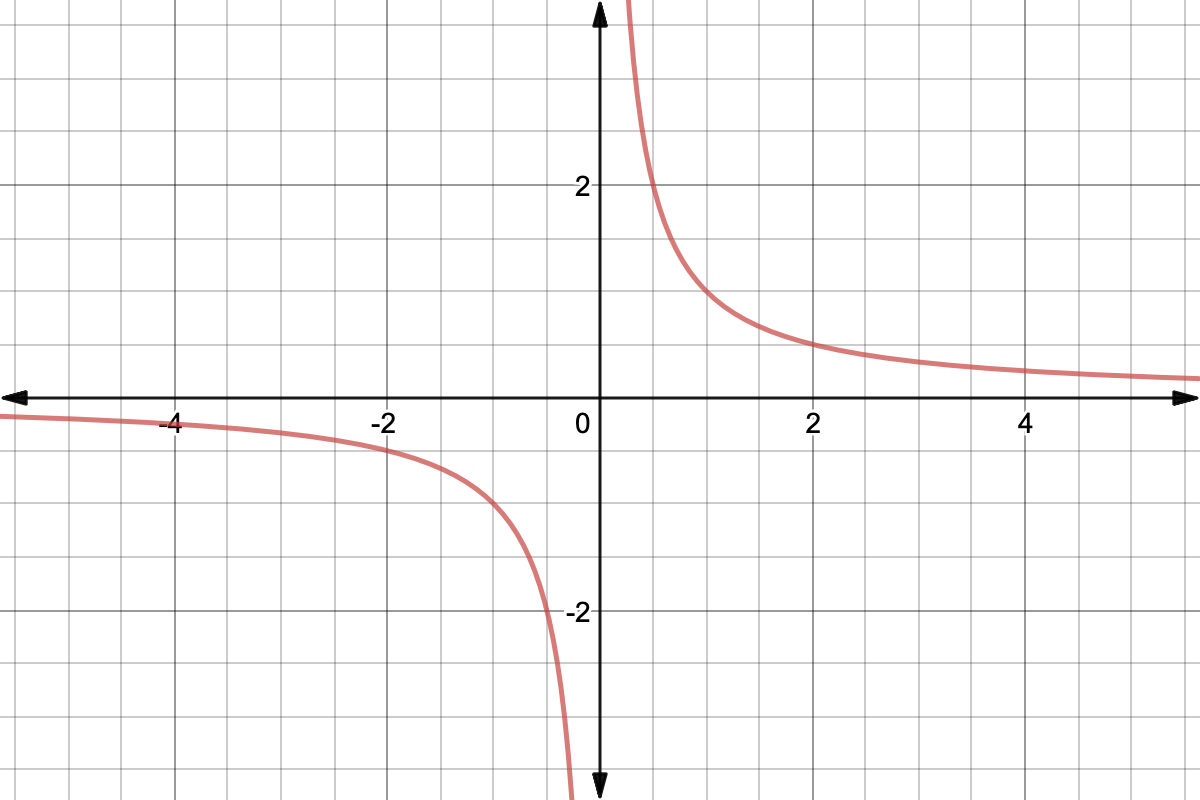



Inversely Proportional Functions In Depth Expii
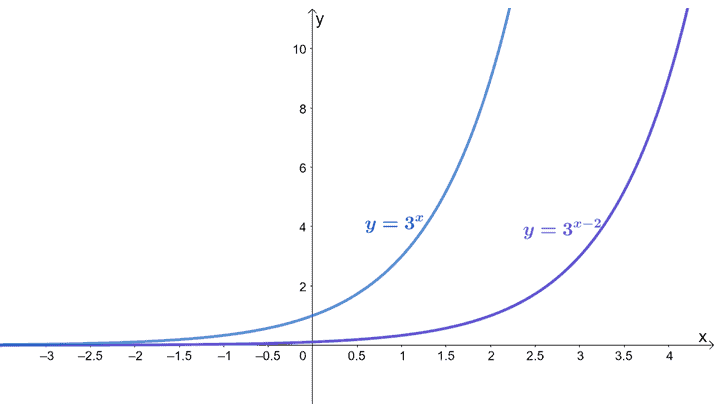



Exponential Function Properties Graphs Applications
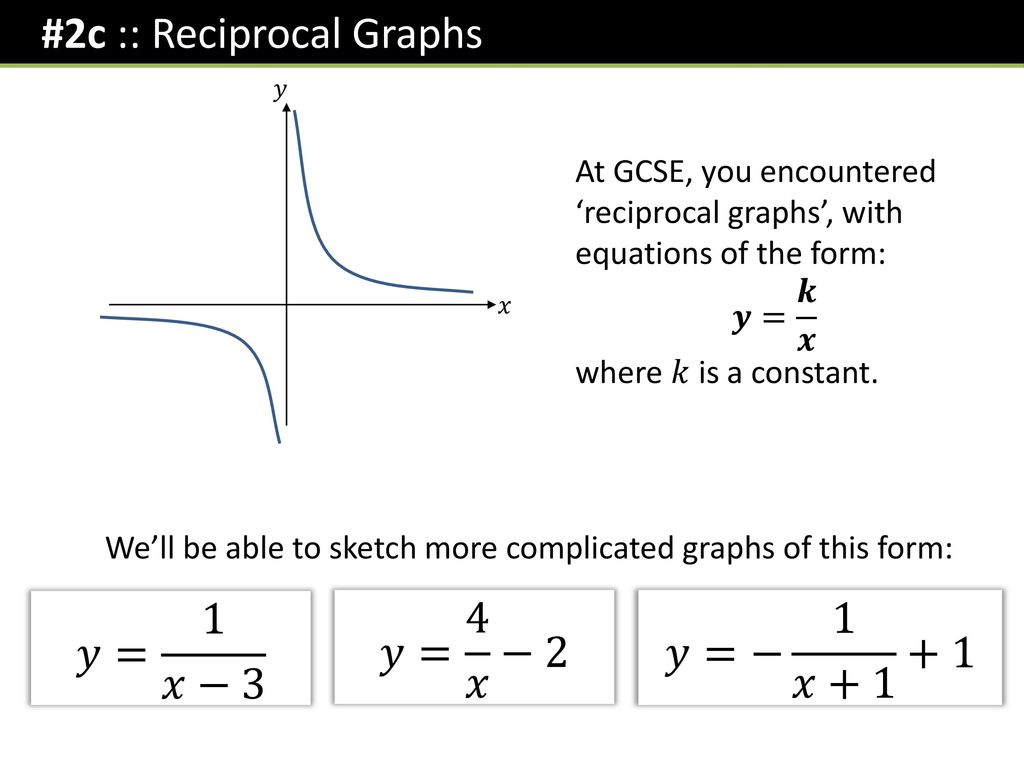



Igcse Fm C1 Sketching Graphs Ppt Download
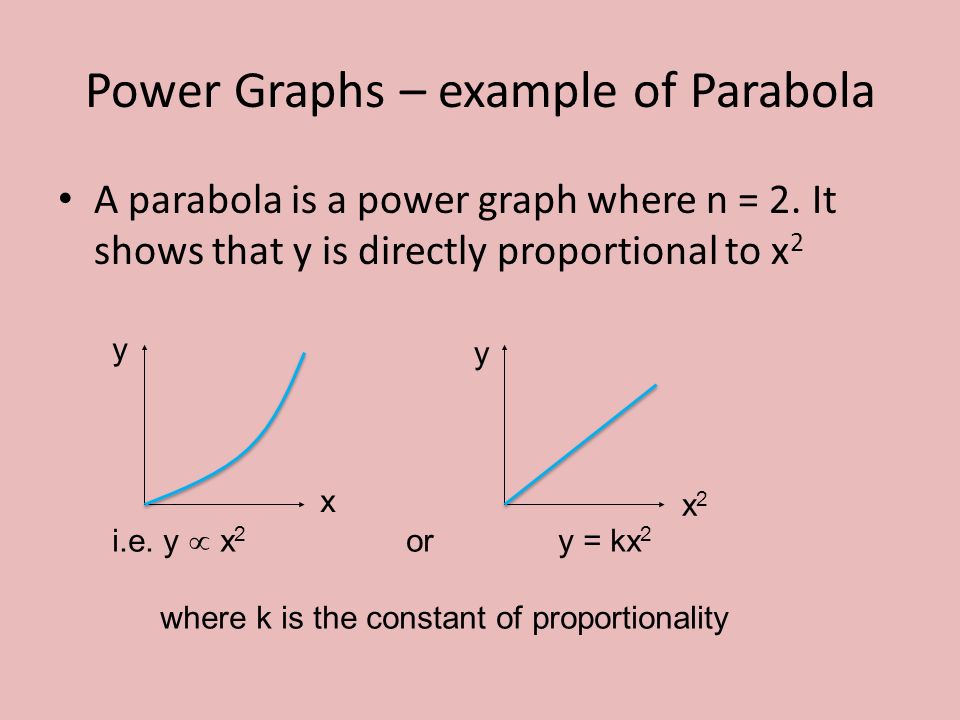



Proportional Techniques Standard Graphs Proportional Techniques Ppt Download
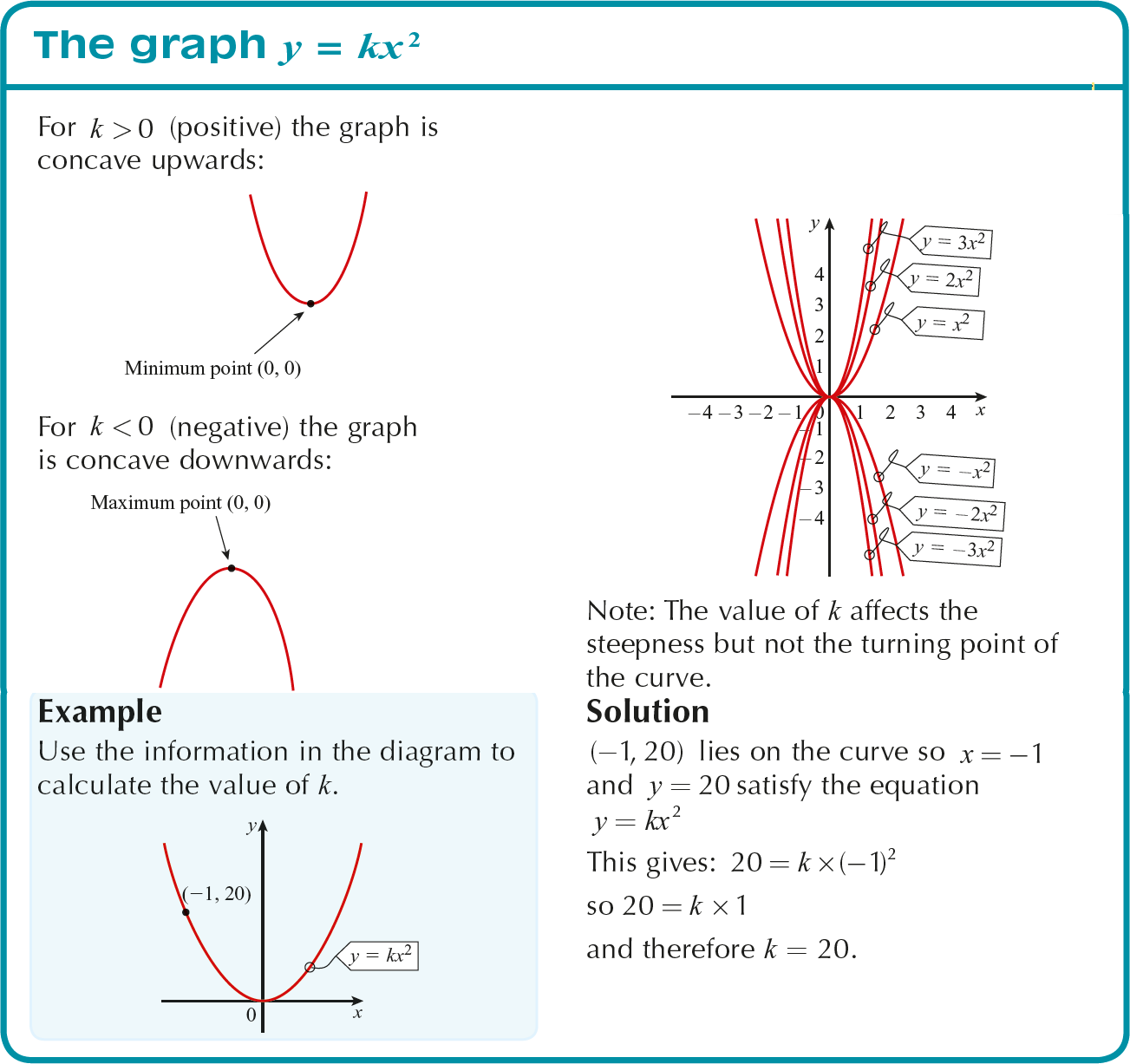



Quadratic Graphs Including Parabolas Sketching Related Graphs And Relationship To Factors



When K 1 Explain How Would You Sketch The Graph When The Given Equation Is In The Form Of Y K X A X B X C Quora




Graphing Of Data Why Do We Display Data




Quadratic Functions Mathnology




Graphing Of Data Why Do We Display Data With Graphs Ppt Download
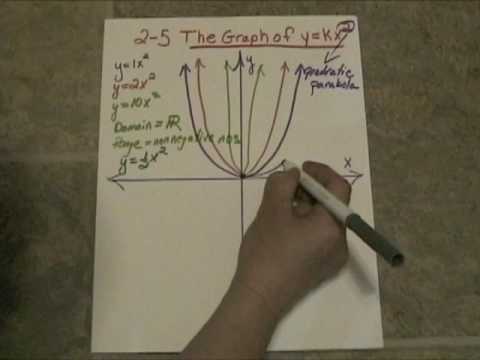



2 5 The Graph Of Y Kx 2 Youtube



Drawing Trigonometric Graphs The Basic Graphs You Should




The Characteristics Of The Graph Of A Reciprocal Function Graphs And Functions And Simultaneous Equations



All Elementary Mathematics Study Guide Functions And Graphs Elementary Functions And Their Graphs



Which Of The Following Graph Represents The Equation Y Kx 2 Sarthaks Econnect Largest Online Education Community




Graphs Of Y 2 Kx Geogebra
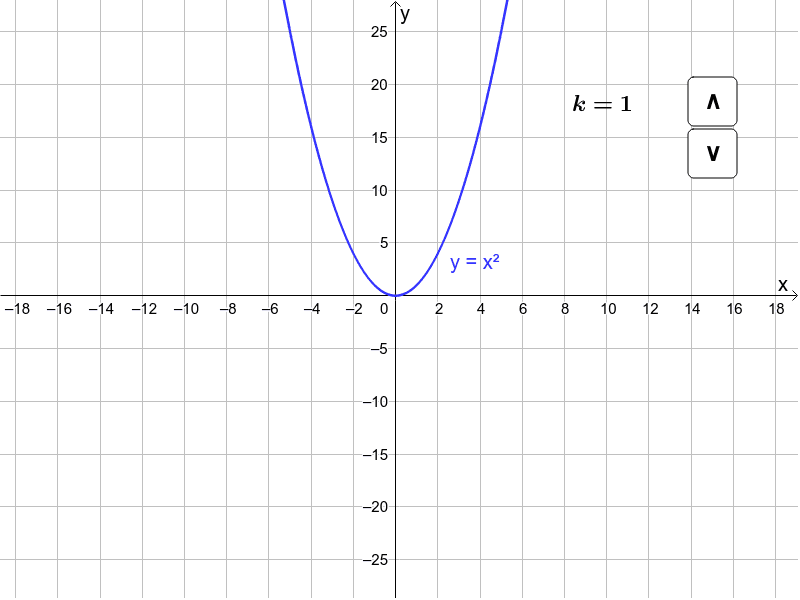



Y Kx 2 Geogebra



Y Kx 2 Parabolic Exponential Proportion Distance Is
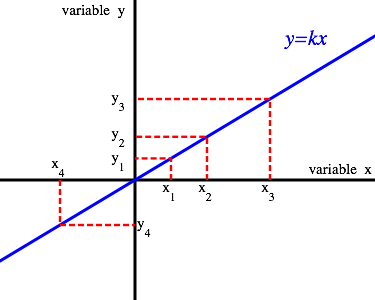



Introduction To Linear Functions Boundless Algebra



Solution Find The Value Of K For Which The Line Y X K Is A Tangent To The Curve Y To The Power Of 2 5x 10



Quadratic Functions




The Characteristics Of The Graph Of A Reciprocal Function Graphs And Functions And Simultaneous Equations
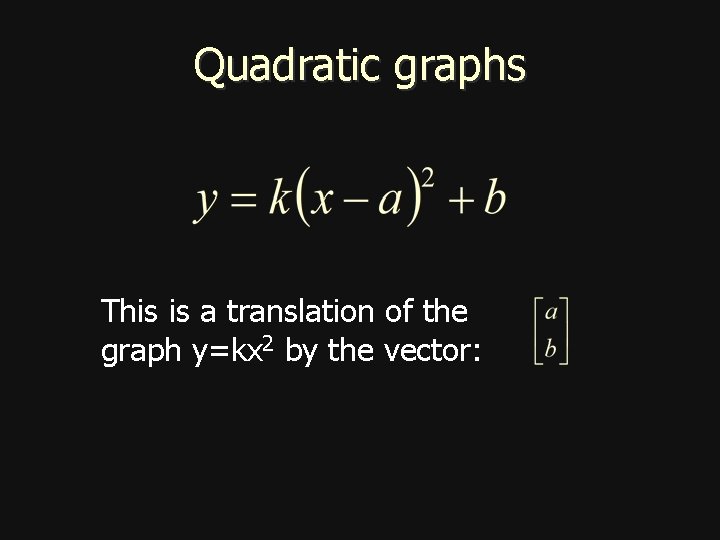



Quadratic Functions3 What Is A Perfect Square How
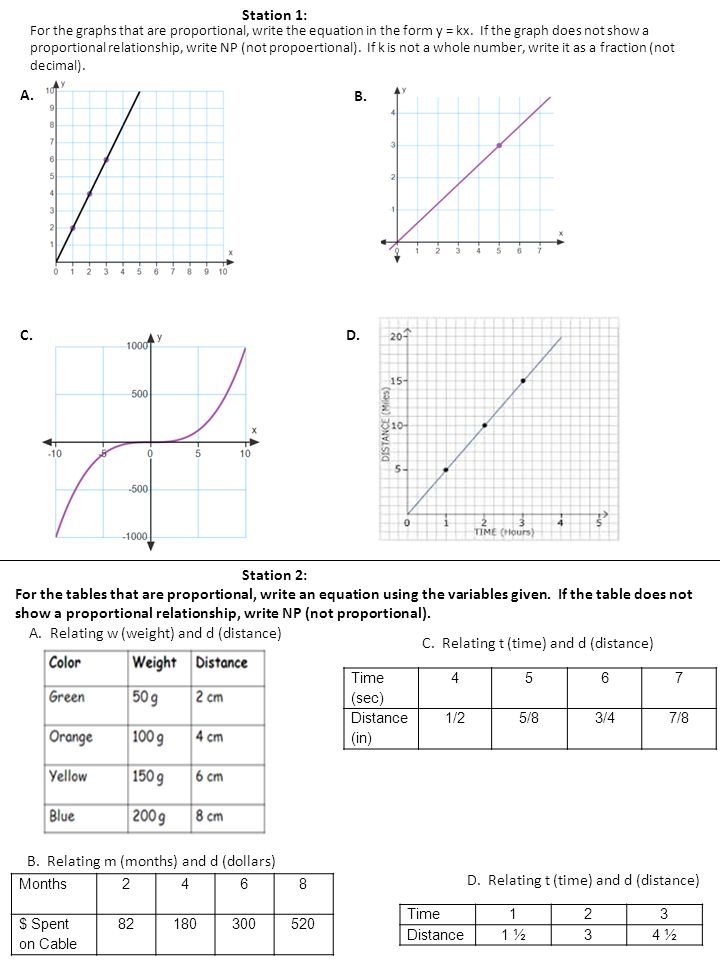



For The Graphs That Are Proportional Write The Equation In The Form Y Kx If The Graph Does Not Show A Proportional Relationship Write Np Not Propoertional Ppt Download
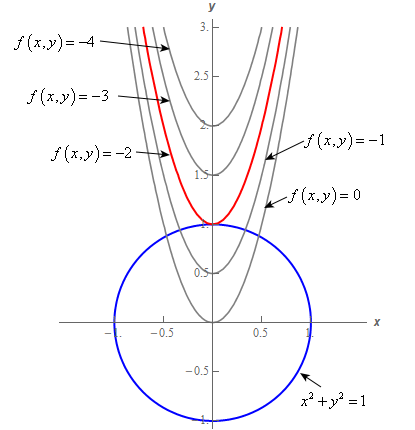



Calculus Iii Lagrange Multipliers
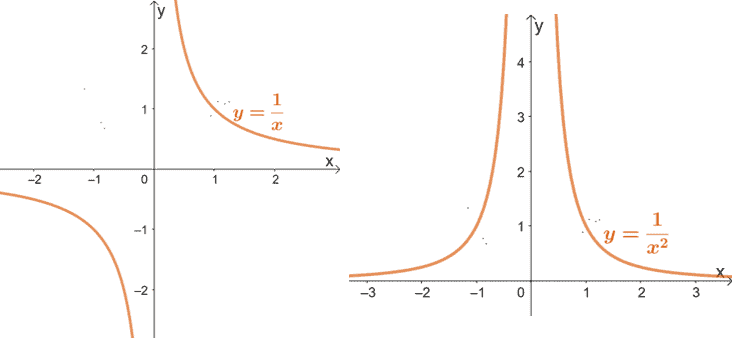



Power Function Properties Graphs Applications



Lesson 4 Parabolas In This Lesson Students Will Become Familiar With The Equations And Graphs Of Parabolas The Definition Of A Parabola Will Be Learned Both Algebraically And Using The Distance Relationship Students Will Learn How To Construct A Parabola




Graphing Exponential Functions
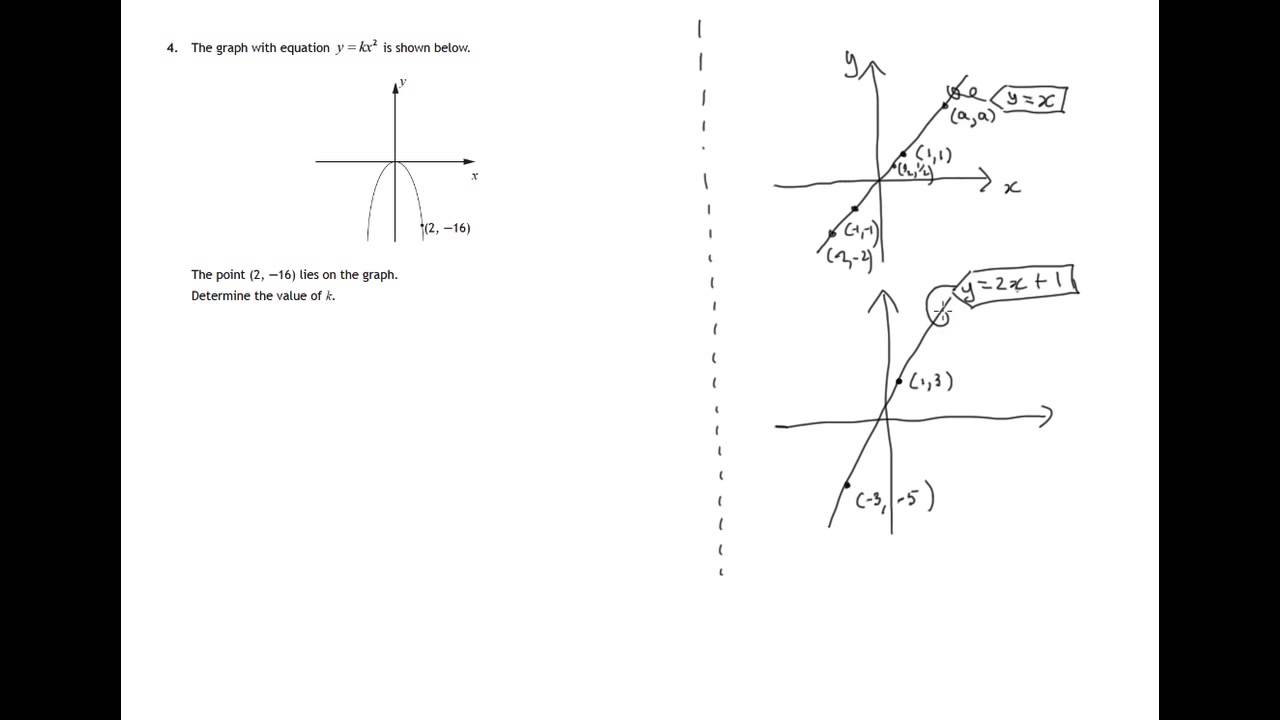



Quadratic Graphs Including Parabolas Sketching Related Graphs And Relationship To Factors
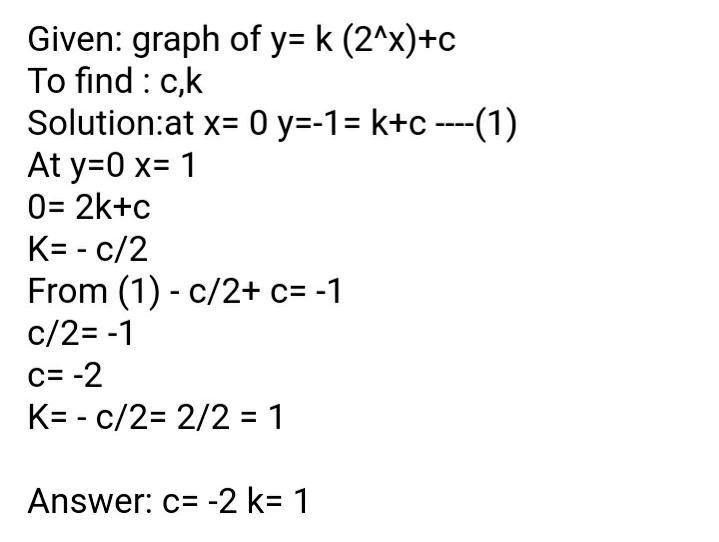



11 The Graph Below Shows The Curve Y K2x C W Gauthmath




Given The Graphs Of Y F X And Y G X Sketch The Graph Of Y K X Mathematics Stack Exchange



What Is The Value S Of K To Make The Equation An Empty Graph If X Y 4x 4y K 17k 24 K 2 Quora




Pendulum Mock Lab Ppt Video Online Download
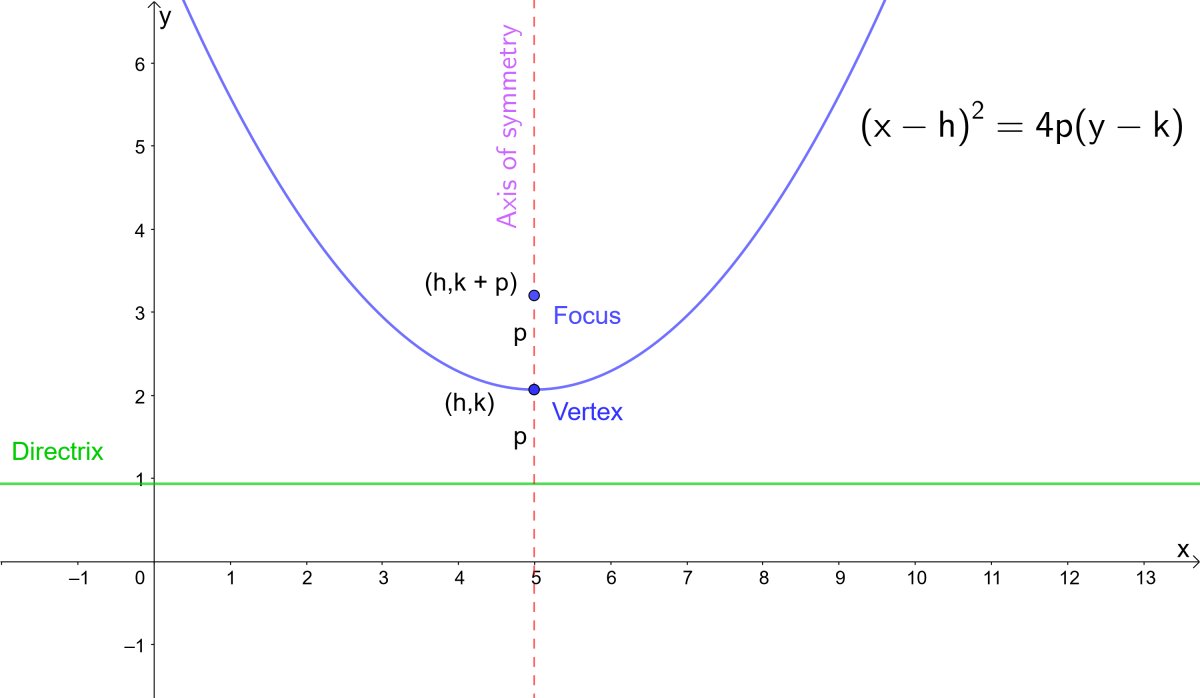



Parabola Equations And Graphs Directrix And Focus And How To Find Roots Of Quadratic Equations Owlcation
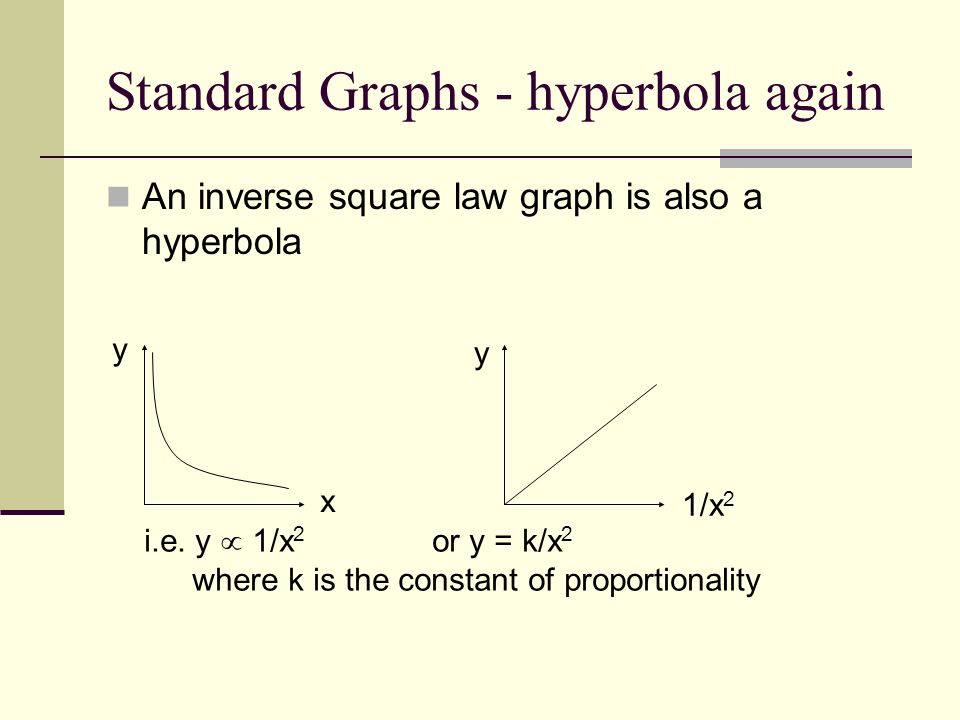



Physics And Physical Measurement Topic 1 2 Measurement And Uncertainties Ppt Download



Http Www Ia Maths Co Uk Site Wp Content Uploads 14 06 Quadratics Notes Pdf




Non Linear Further Maths U3 4




Graphs Of Functions In The Form Y Kx N Youtube




Find The Values Of K For Which The Graph Of Y Kx 3 Gauthmath
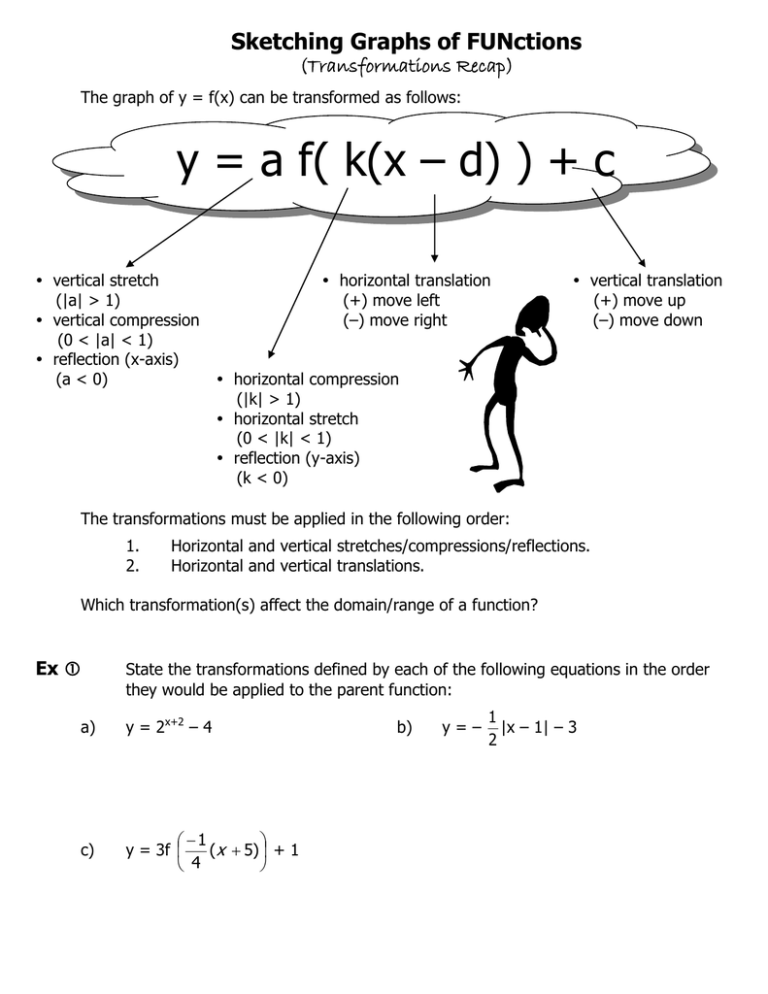



Y A F K X D C



Search Q Inverse Square Graph Tbm Isch



Pplato Basic Mathematics Quadratic Functions And Their Graphs
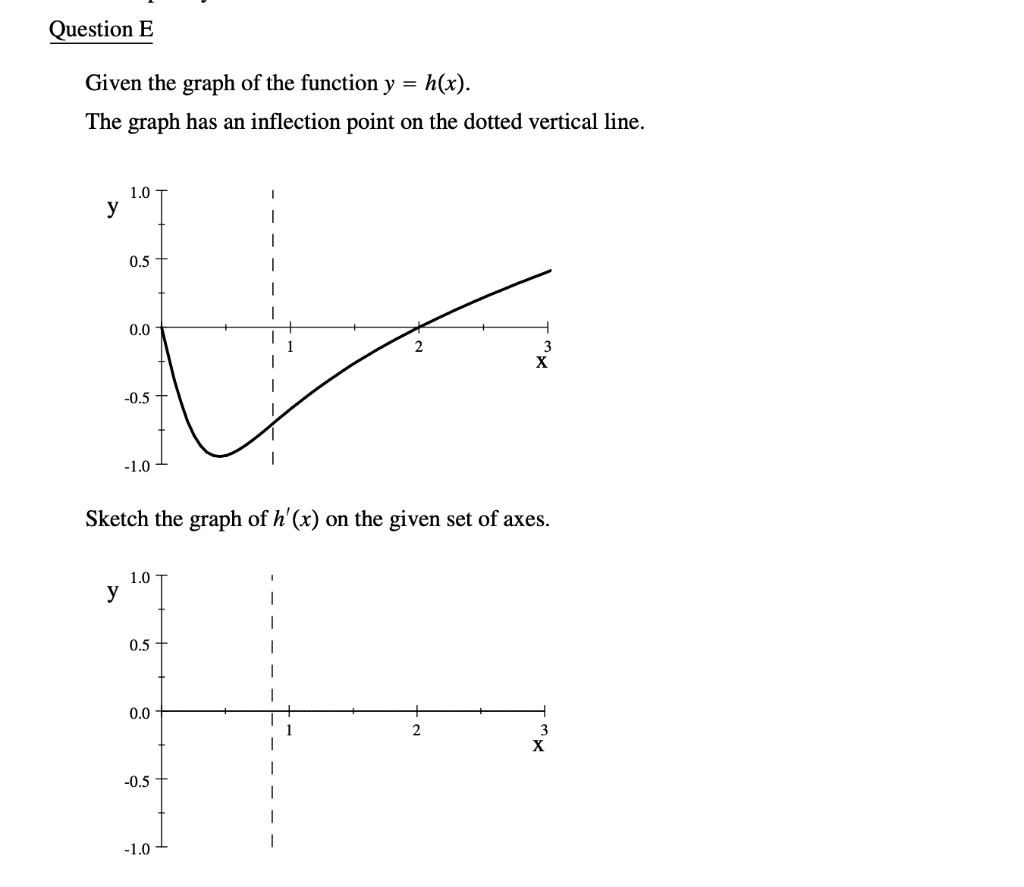



Question D The Function Y K X Is Graphed Below U Chegg Com
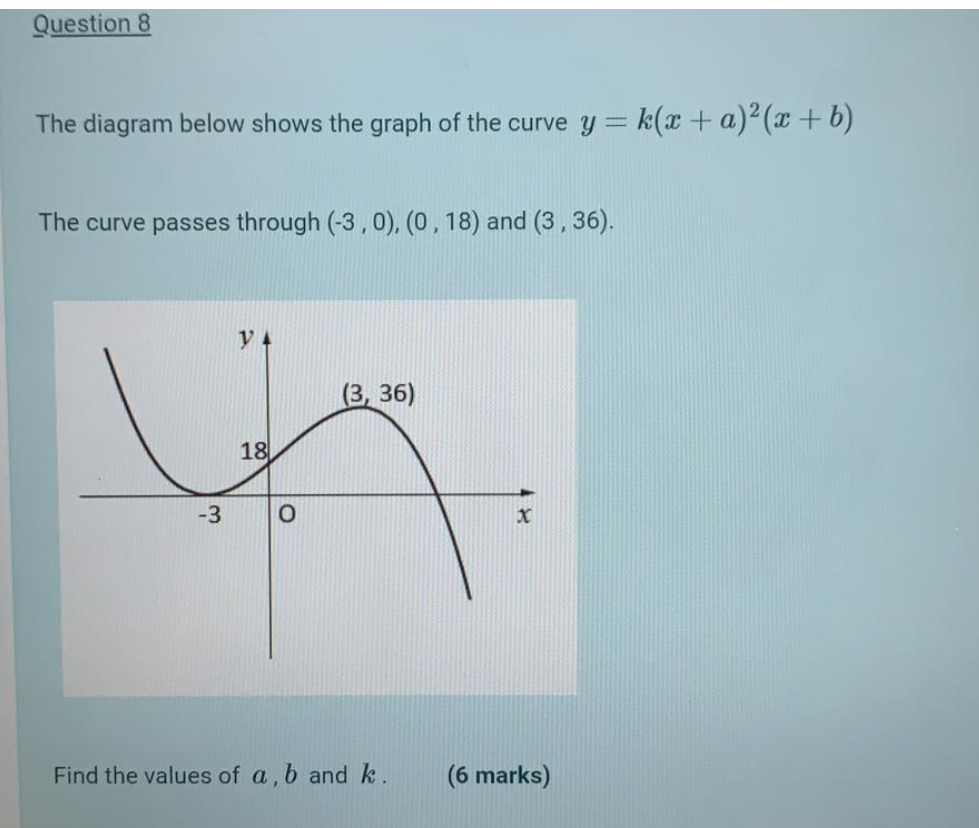



Question 8 The Diagram Below Shows The Graph Of The Chegg Com




Drawing Graphs Shapes Cie Igcse Maths Revision Notes
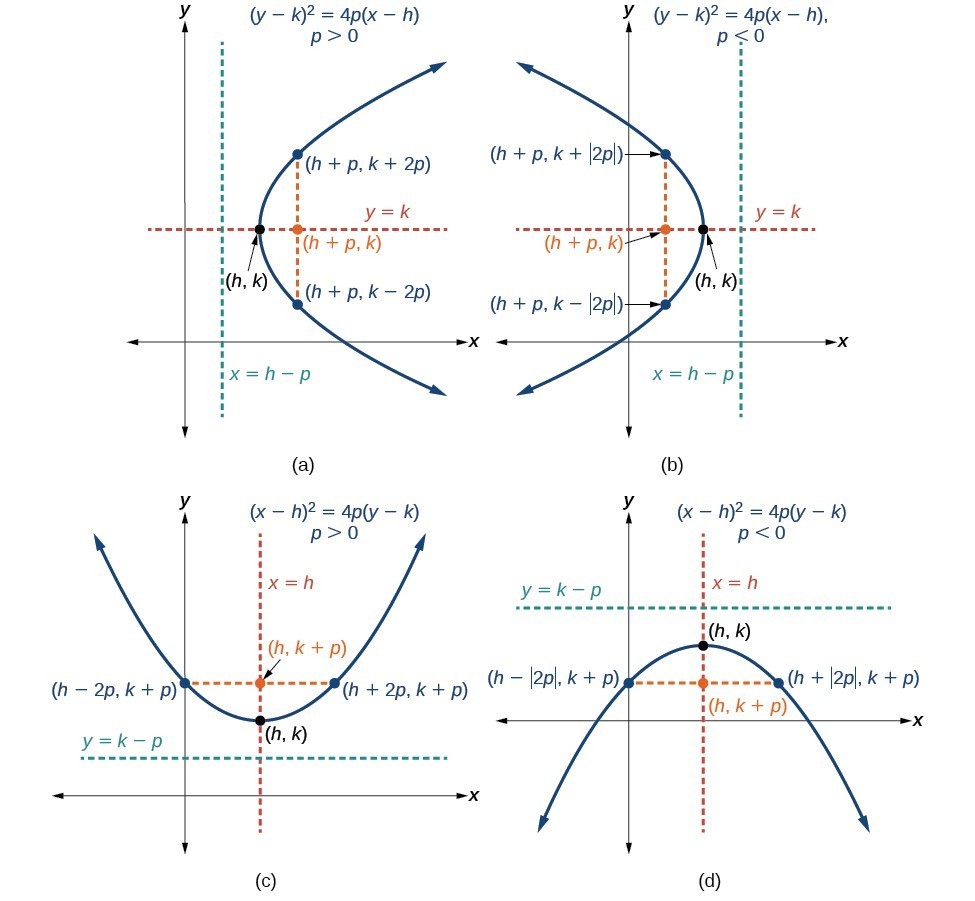



Parabolas With Vertices Not At The Origin College Algebra




2 Let F X Ksin Kx Where K Is A Positive Constant Find The Area Of The Region Bounded By One Arch Of The Graph Of F And The X Axis Study Com




Explore Y K X Inverse Variation
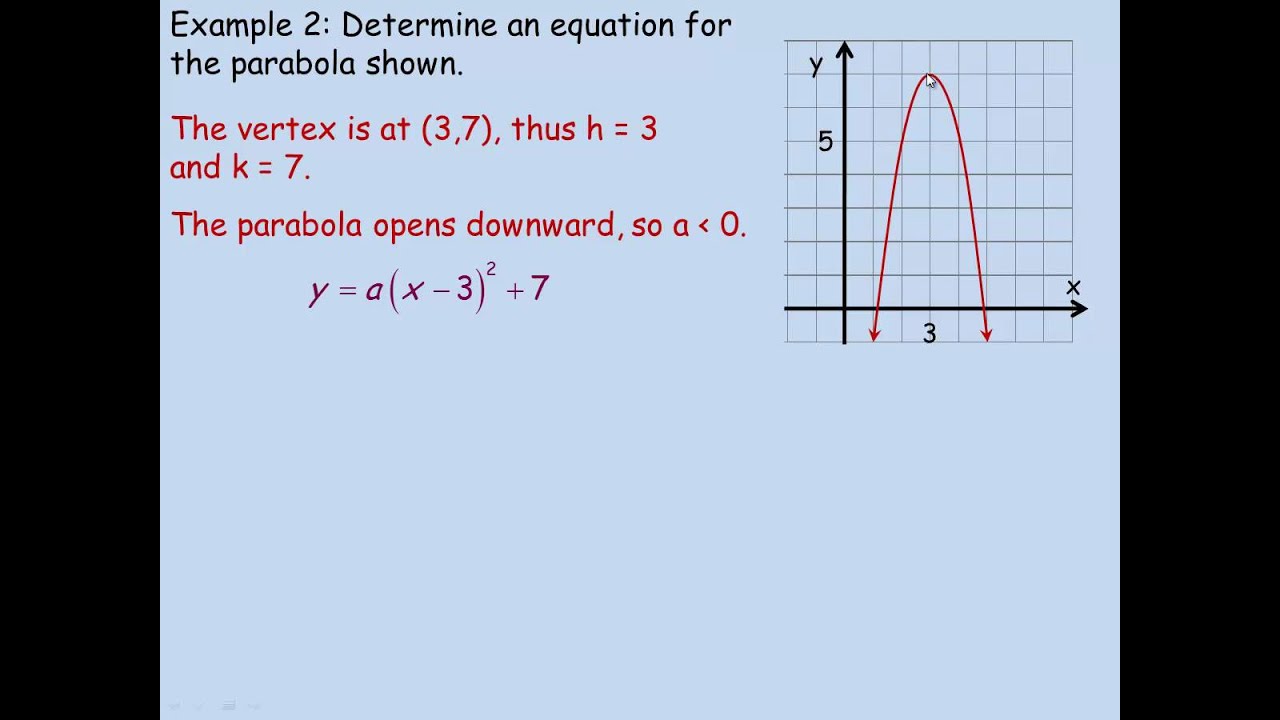



Graphing Y A X H K Youtube



Graphatron 1 10 Graphs And Equations



Graphing Quadratic Functions



Http Www Ia Maths Co Uk Site Wp Content Uploads 14 06 Quadratics Notes Pdf
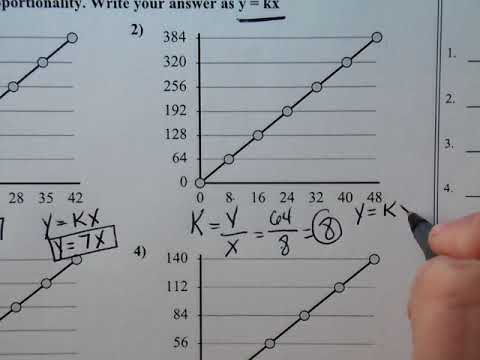



Writing The Equation Of Graphs With The Constant Of Proportionality Y Kx Youtube
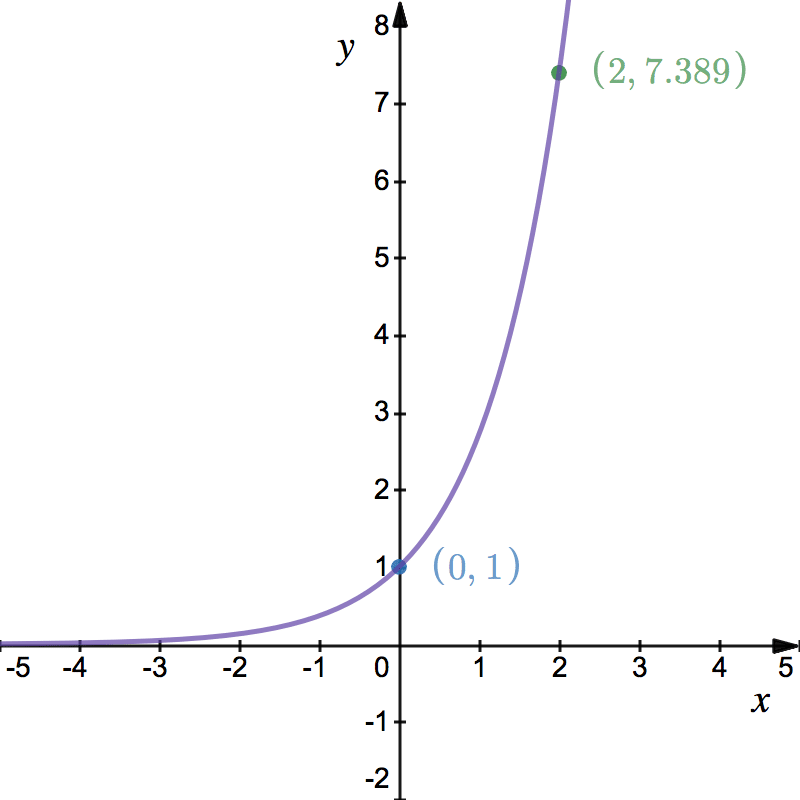



Differentiating E To The Kx Studywell



Graph Of Scfc Plate P A B Note Y K And X Aspect Ratio P Fig 6 Download Scientific Diagram




An Explanation Of Direct And Inverse Proportion Helpful Colin
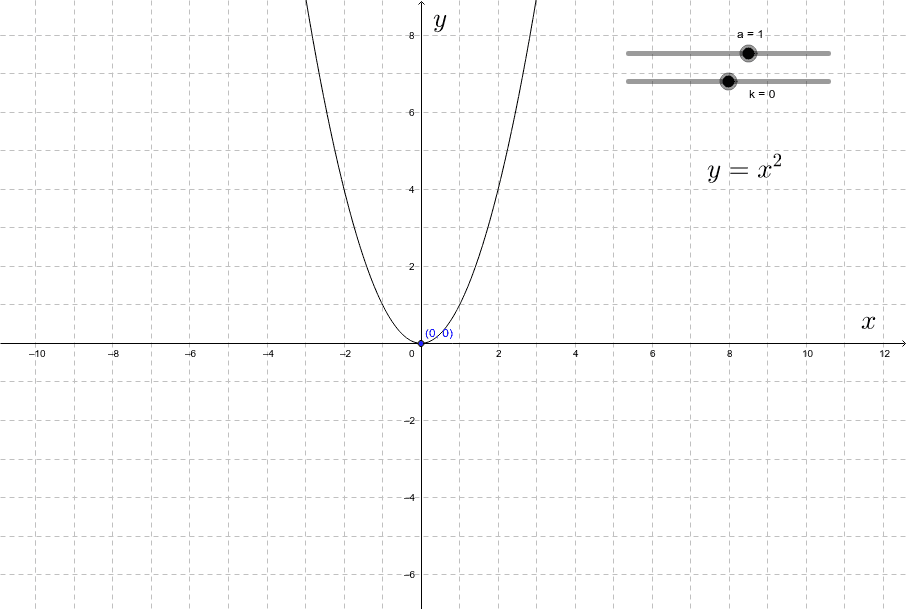



Graph Of Y Ax 2 K Geogebra



Quadratics Graphing Parabolas Sparknotes




Graph Of Y K X By Anna Wasielewski



1




How To Solve The Following Question Find The Values Of K For Which The Line Y Kx 2 Meets The Curve Y 2 4x X 2 Quora
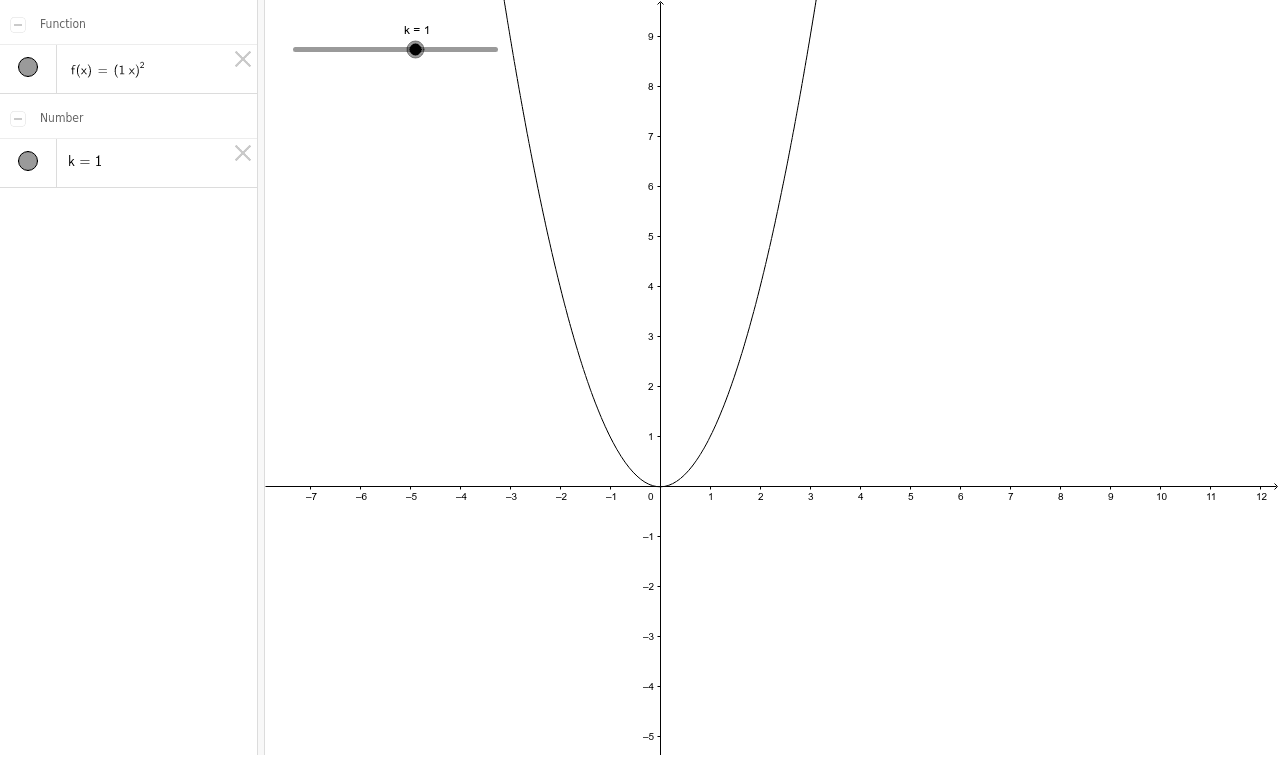



Describing Variation Graphs Station 2 The Graph Of Y Kx 2 Geogebra



Exponetials And Growth Functions




Graph Of Cscf Plate P A B Note Y K And X Aspect Ratio P Fig 3 Download Scientific Diagram




Graph Of Scfc Plate P A B Note Y K And X Aspect Ratio P Download Scientific Diagram




Non Linear Further Maths U3 4



Bennett Knapek 3 4 Home




Determine The Value Of K Such That The Line Determined By The Points 3 2 And 1 2 Is Tangent To The Graph Of Y Frac K X 1 Mathematics Stack Exchange



0 件のコメント:
コメントを投稿